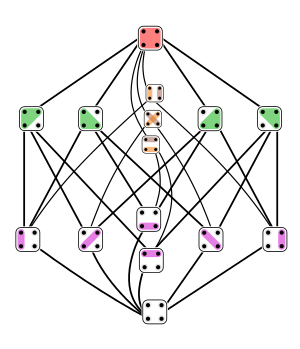
Partition of a set
|
Read other articles:

Kerinyu limau Aloysia citrodora TaksonomiDivisiTracheophytaSubdivisiSpermatophytesKladAngiospermaeKladmesangiospermsKladeudicotsKladcore eudicotsKladasteridsKladlamiidsOrdoLamialesFamiliVerbenaceaeTribusLantaneaeGenusAloysiaSpesiesAloysia citrodora Paláu, 1784 lbs Aloysia citrodora, kerinyu limau, adalah spesies tumbuhan berbunga dalam keluarga kerinyu Verbenaceae, yang berasal dari Amerika Selatan. Nama umum lainnya termasuk semak lebah limau . [1] Minyak ini dibawa ke Eropa oleh Spany…

本條目存在以下問題,請協助改善本條目或在討論頁針對議題發表看法。 此條目需要补充更多来源。 (2018年3月17日)请协助補充多方面可靠来源以改善这篇条目,无法查证的内容可能會因為异议提出而被移除。致使用者:请搜索一下条目的标题(来源搜索:羅生門 (電影) — 网页、新闻、书籍、学术、图像),以检查网络上是否存在该主题的更多可靠来源(判定指引)。 此�…

Gerard Hoet, Nubuat Ahia kepada Yerobeam, 1728. Ahia orang Silo (Ibrani: אחיה השילוני, Aḥiya[1] ('saudara laki-laki Yah'[2]) Hashiloni); Inggris: Ahijah the Shilonitecode: en is deprecated ), adalah seorang nabi keturunan Lewi yang berasal dari kota Silo, yang hidup pada zaman rajaSalomo, seperti yang disebutkan dalam Kitab 1 Raja-raja pada Alkitab Ibrani. Ahia meramalkan bahwa Yerobeam bin Nebat akan menjadi raja (1 Raja–raja 11:29).[3] Warisan Ibrani …

Pour les articles homonymes, voir Fromantin. Jean-Christophe Fromantin Jean-Christophe Fromantin en 2015. Fonctions Vice-président du conseil départemental des Hauts-de-Seinechargé des Infrastructures routières et navigables En fonction depuis le 1er juillet 2021(2 ans, 9 mois et 2 jours) Président Georges Siffredi Prédécesseur Jean-Didier Berger Conseiller départemental des Hauts-de-Seine En fonction depuis le 1er juillet 2021(2 ans, 9 mois et 2 jours) Avec…

العلاقات الدنماركية البليزية الدنمارك بليز الدنمارك بليز تعديل مصدري - تعديل العلاقات الدنماركية البليزية هي العلاقات الثنائية التي تجمع بين الدنمارك وبليز.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية للدولتين: وجه المقارنة ا�…

Election in New Jersey Main article: 1996 United States presidential election 1996 United States presidential election in New Jersey ← 1992 November 5, 1996 2000 → Nominee Bill Clinton Bob Dole Ross Perot Party Democratic Republican Independent Home state Arkansas Kansas Texas Running mate Al Gore Jack Kemp Pat Choate Electoral vote 15 0 0 Popular vote 1,652,329 1,103,078 262,134 Percentage 53.72% 35.86% 8.52% County Results Clinton 40…

Ofra HazaHaza pada 1994LahirBat-Sheva Ofra Haza(1957-11-19)19 November 1957Hatikva, Tel Aviv, IsraelMeninggal23 Februari 2000(2000-02-23) (umur 42)Ramat Gan, IsraelSebab meninggalPneumonia terkait AIDSMakamPemakaman YarkonPekerjaan Penyanyi penulis lagu pemeran Tahun aktif1969–2000Suami/istriDoron Ashkenazi (m. 1997)Karier musikGenre Musik dunia pop elektronika etnis Musik Timur Tengah synthpop Instrumen Vokal piano Label Hed Arzi EastWest Shanachi…

Karakara Utara (Caracara cheriway) di Kosta Rika Karakara Berjambul adalah nama Falconiformes falconid. Karakara Berjambul terdiri dari: Karakara Utara, Caracara cheriway Karakara Selatan, Caracara plancus Karakara Guadalupe, Caracara lutosa Artikel bertopik hewan ini adalah sebuah rintisan. Anda dapat membantu Wikipedia dengan mengembangkannya.lbs

American baseball player and executive (1936–2022) Baseball player Lee ThomasFirst baseman / Right fielderBorn: (1936-02-05)February 5, 1936Peoria, Illinois, U.S.Died: August 31, 2022(2022-08-31) (aged 86)St. Louis, Missouri, U.S.Batted: LeftThrew: RightMLB debutApril 22, 1961, for the New York YankeesLast MLB appearanceSeptember 27, 1968, for the Houston AstrosMLB statisticsBatting average.255Home runs106Runs batted in428 Teams New York Yankees (1961) Los An…

Fusi orari dell'Europa: Azzurro Western European Time (UTC+0) Blu Western European Time (UTC+0)Western European Summer Time (UTC+1) Rosso Central European Time (UTC+1)Central European Summer Time (UTC+2) Giallo Ora di Kaliningrad (UTC+2). Ocra Eastern European Time (UTC+2)Eastern European Summer Time (UTC+3) Verde Ora di Mosca (UTC+3) I colori più chiari indicano i paesi che non osservano l'ora legale Eastern European Summer Time (EEST) è la denominazione del fuso orario dell'Europa orientale …

Синелобый амазон Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлада:ЗавропсидыКласс:Птиц�…

For other songs, see High school (disambiguation). 2013 single by Nicki Minaj featuring Lil WayneHigh SchoolSingle by Nicki Minaj featuring Lil Waynefrom the album Pink Friday: Roman Reloaded – The Re-Up ReleasedApril 16, 2013 (2013-04-16)Recorded2012Genre Hip hop R&B Length3:38Label Young Money Cash Money Republic Songwriter(s) Onika Maraj Dwayne Carter Matthew Samuels Tyler Williams Producer(s) Boi-1da T-Minus Nicki Minaj singles chronology Tapout (2013) High School (2…

Liga 1 1.Musim2021–2022Tanggal27 Agustus 2021 – 31 Maret 2022JuaraBali UnitedPertandingan perdanaBali United 1–0 Persik(27 Agustus 2021)DegradasiPersipuraPerselaPersirajaPiala AFC 2022Bali UnitedPSM MakassarLiga Champions AFC 2023–24Bali United[cat. 1]Jumlah pertandingan306Jumlah gol735 (2,4 per pertandingan)Pencetak golterbanyakIlija Spasojević(22 gol)Kemenangan kandangterbesarBali United 5-0 Persiraja(30 November 2021)Persikabo 1973 5-0 Persiraja(9 Desember 2021)Kemenanga…

The Royal Dragoon GuardsRegimental badgeActive1 August 1992 – presentAllegiance United KingdomBranch British ArmyTypeLine cavalryRoleArmoured cavalrySizeOne regimentPart ofRoyal Armoured CorpsGarrison/HQRHQ: YorkRegiment: Battlesbury Barracks, WarminsterNickname(s)“The First and Last” and “Irish & Yorks Cavalry”Motto(s)Quis SeparabitWho shall separate usMarchQuick – Fare Thee Well InniskillingSlow – 4th Dragoon Guards (first two themes) + 7th Dragoon Guards (firs…

1964 film by B. R. Panthulu Muradan MuthuTheatrical release posterDirected byB. R. PanthuluScreenplay byM. S. SolaimalaiProduced byB. R. PanthuluStarringSivaji GanesanDevikaCinematographyV. RamamurthiEdited byR. DevarajanMusic byT. G. LingappaProductioncompanyPadmini PicturesRelease date 3 November 1964 (1964-11-03) CountryIndiaLanguageTamil Muradan Muthu (transl. Rogue Muthu) is a 1964 Indian Tamil-language film, directed and produced by B. R. Panthulu. The film stars Sivaj…

Monsters & Co.Mike, Sulley e Boo in una scena del filmTitolo originaleMonsters, Inc. Lingua originaleinglese Paese di produzioneStati Uniti d'America Anno2001 Durata92 min Rapporto1,85:1 Genereanimazione, commedia, fantastico, avventura RegiaPete Docter co-regia: Lee Unkrich, David Silverman SoggettoPete Docter, Jill Culton, Jeff Pidgeon, Ralph Eggleston SceneggiaturaAndrew Stanton, Daniel Gerson ProduttoreDarla K. Anderson Produttore esecutivoJohn Lasseter, Andrew Stanton Casa d…

Italian football club For the Spanish club with the same name, see SP Villafranca. Football clubVillafrancaFull nameAssociazione Sportiva Dilettantistica VillafrancaNickname(s)Cappe Falzo Hasa DalFounded1920GroundStadio Comunale,Villafranca di Verona, ItalyChairmanMirko CordioliManagerPaolo CorghiLeagueEccellenza2019–20Serie D/B, 20th (relegated) Home colours Away colours Associazione Sportiva Dilettantistica Villafranca is an Italian association football club, based in Villafranca di Verona, …

提示:此条目页的主题不是中華人民共和國最高領導人。 中华人民共和国 中华人民共和国政府与政治系列条目 执政党 中国共产党 党章、党旗党徽 主要负责人、领导核心 领导集体、民主集中制 意识形态、组织 以习近平同志为核心的党中央 两个维护、两个确立 全国代表大会 (二十大) 中央委员会 (二十届) 总书记:习近平 中央政治局 常务委员会 中央书记处 中�…

拉尔·巴哈杜尔·夏斯特里第二任印度总理任期1964年6月9日—1966年1月11日总统薩瓦帕利·拉達克里希南前任古爾扎里拉爾·南達继任古爾扎里拉爾·南達印度外交部長任期1964年6月9日—1964年7月18日总理自己前任古爾扎里拉爾·南達继任斯瓦倫·辛格(英语:Swaran Singh)印度內政部長任期1961年4月4日—1963年8月29日总理賈瓦哈拉爾·尼赫魯前任戈文德·巴拉布·潘特(英语:Govind Ballabh …
2020年夏季奥林匹克运动会波兰代表團波兰国旗IOC編碼POLNOC波蘭奧林匹克委員會網站olimpijski.pl(英文)(波兰文)2020年夏季奥林匹克运动会(東京)2021年7月23日至8月8日(受2019冠状病毒病疫情影响推迟,但仍保留原定名称)運動員206參賽項目24个大项旗手开幕式:帕维尔·科热尼奥夫斯基(游泳)和马娅·沃什乔夫斯卡(自行车)[1]闭幕式:卡罗利娜·纳亚(皮划艇)[2…









![{\displaystyle [a]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ea82bc70a8e322f13a3c4e5b9d5d69e8ef097ad8)




![{\displaystyle a\in [b]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8abb5047c33633d5a0697408f3df2aead151e4ea)
![{\displaystyle b\in [a]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/cd1c441d4221216c8947ac50d43331042f72672b)